Hotelling's Model
Suppose that two owners of refreshment stands, George and Henry, are trying to decide where to locate along a stretch of beach. Suppose further that there are 100 customers located at even intervals along this beach, and that a customer will buy only from the closest vendor. Finally, assume that the beach is short enough so that total sales are independent of where the vendors locate.
Suppose that initially the vendors locate at points A and C in the illustration below. These locations would minimize the average traveling costs of the buyers and would result in each vendor getting one half of the business. However, this solution would not be an equilibrium. If George moved from point A to point B, he would keep all customers to his left and get some of Henry's customers. For similar reasons, Henry would move toward the center, and in equilibrium, both vendors would locate together in the middle.
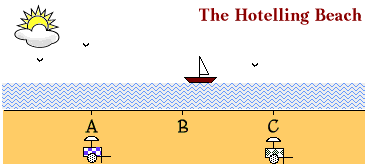
This story of the beach was first told in 1929 by Harold Hotelling and is called Hotelling's model. Although it can give some insights into businesses decisions concerning location and product characteristics, the model has been more useful in explaining certain political phenomena. Instead of two refreshment stands along a beach trying to attract dollars from customers, consider two political candidates along the political spectrum trying to attract votes from voters. Only the candidate who attracts the most votes will win, and a candidate must locate nearer to more voters than his opponent to attract votes. With these rules, there is a strong tendency for each candidate to move to the middle.
In American politics this tendency has a predictable consequence for presidential candidates, who must "sell" on two beaches. To gain the nomination, the candidate must position himself in the middle of the party. Because the average Democrat has significantly different views than the average Republican, Republican and Democratic candidates sound quite different before nominations are decided. After the party nominations are determined, the two candidates must "sell" to the same beach. Republican candidates move to the left and Democratic candidates move to the right. By election time, their positions on issues often seem close enough to many voters so that factors such as personality emerge as keys to the election.
There have been some notable exceptions to this pattern. In 1964, Barry Goldwater won the Republican nomination standing well to the right of the average voter, and was unable or unwilling to reposition himself in the center. In 1972, George McGovern won the Democratic nomination standing well to the left of the average voter, and was unable or unwilling to reposition himself. Both lost in landslides.
A problem with the Hotelling model when applied to commerce is that the results are very sensitive to the cost assumption. There must be some cost to traveling because customers prefer the closest vendor. But these costs must be small, because the people at the end of the beach continue to buy the same amount no matter how far they are from the nearest vendor. If traveling costs are less, then people might not care whether they go to the nearest vendor. If they are greater—so that when the vendor gets far away, people do not bother to go—the vendors will no longer cluster at the middle.
Suppose that the beach is a long beach, and people more than 1000 feet away from any seller buy nothing. Also assume that the beach is 4000 feet long, and the two vendors start at the middle. Originally George sells to customers located from the 1000-foot mark to the middle at 2000 feet, and Henry sells from 2000 feet to 3000 feet. If George moves to the 1000-foot mark, he will gain 1000 feet of new territory, and he will lose only 500 feet to Henry. At the 1000-foot mark, he will sell to all people from 0 to 1000 feet. He will also sell to those people between him and Henry who are closer to him. Because Henry did not move, but stayed at the 2000-foot mark, George will get all the customers up to the 1500-foot mark. Equilibrium in this case will occur only when Henry moves to the 3000-foot mark.
In Hotelling's original model with small traveling costs, location decisions were not economically efficient. By increasing traveling costs, it seems that we can have location decisions that are economically efficient. However, the next section shows that adding transport costs results in new efficiency problems.
Copyright Robert Schenk